On-line Math 21

On-line Math 21

2.3  Higher Derivatives

The second derivative of a function f , written f¢¢, is defined to be
f¢¢(x): = ( f¢(x)) ¢.
It's just the derivative of the derivative of f .

It is also written in a number of different ways, if f(x) = y :
f¢¢(x) = d2f
dx2
 = d2y
dx2
 = D2(f) = D2(y) = f(2) = fxx.

Example 1 Find the following derivative:
( sin(x)) ¢¢.

Solution

Exercise 1 Find the second derivative
æ
è
Ö
 
x2+2x-1
 
 ö
ø 		¢¢

Answer

Exercise 2 Find the indicated third derivative:
( x2+4x+1) ¢¢¢

Answer

The third derivative of f(x) , f¢¢¢(x) , is defined by
f¢¢¢(x) : = ( f¢¢(x)) ¢.

Email Address (Required to submit answers):

I'll leave to you to guess how to denote third derivatives. As to what a third derivative means, it's actually not that clear. But we haven't yet discussed what the second derivative means.

2.3.1  Yeah, so what does the second derivative mean?

You can easily see what the first derivative of a function f means, in terms of the slope of the curve, or the speed of motion. The second derivative f¢¢ is a bit more subtle. It measures the slope of f¢, and since the slope of f¢ determines how f¢ is changing (increasing, decreasing, etc.), then f¢¢ tells you how the slope is changing. You can see that in the graph; in a region where f¢¢(x) > 0 , the curve will be ``curving'' up (or concave up).

On the other hand, in a region where f¢¢(x) < 0 , the curve will be curving downward (or concave down).

I want to emphasize that it is possible for f(x) to be decreasing f¢(x) < 0 and concave up f¢¢(x) > 0 , as well as any other combination of the two phenomena.

A common way that we use f¢¢(x) casually is given by the line ``The rate of increase of unemployment is slowing''. This does not say that more people are employed, but that the number of unemployed is growing more slowly than before.

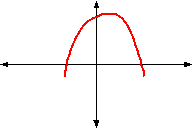
But the real meaning of the second derivative f¢¢(x) relates more to motion. If the position of a particle at time t is given by s(t) , then we saw that its velocity was v = s¢(t) . The second derivative of the position, the rate of change of the velocity, is the acceleration
a(t) = v¢(t) = s¢¢(t).
The standard examples of a ball being thrown up in the air, with a position of, say, s(t) = -16t2+40t+5 , reflect the standard elementary physics idea that, without air, all falling objects (near the surface of the Earth) will have a constant acceleration of 32  ft/(sec)2 , straight down. For this example:
s(t)
=
-16t2+40t+5
v(t) = s¢(t)
=
-32t+40, and
a(t) = v¢(t) = s¢¢(t)
=
-32,
in feet per second (the negative value indicates downward acceleration).

Example 2 Egbert tosses a baseball straight up, and measures its position at each time t . He finds that the position s(t) (in feet above his hand) at time t seconds t is
s(t) = -16t2+24t+7.
Find the velocity and acceleration at time t = 0 , when he let go of the ball.

Solution

[Idea for an interactive thing. The user chooses a number a which gives the initial velocity, and the animation then runs through the position at time t according to s = -16t2+v0t with appropriate scale.] Text: Choose a value for the initial velocity v0 . Pressing the ``toss'' button will show what happens when you toss a ball upward from its rest position with that initial velocity (velocity at t = 0 ). v0 should be between 1 and 40, so that you don't break anything.

Copyright (c) 2000 by David L. Johnson.

File translated from TEX by TTH, version 2.61.
On 9 Nov 2000, 22:51.