On-line Math 21

4.5  Curve Sketching

Example 8

y = xe1/x.

Solution

   Asymptotes

As x® 0 , this function gets a little complicated. For x > 0 , yet x near 0, this one-sided limit

lim x® 0+  xe1/x

is an <a href="hospital.html»indeterminate form</a>, of type 0·¥. In order to convert this to something that l'Hôpital's rule can handle, it needs to be switched to either ¥/¥ or 0/0 . Since I don't want to invert the exponential term, we'll convert to ¥/¥:

lim x® 0+  xe1/x = lim x® 0+  e1/x 1/x = lim x® 0+  e1/x(-1/x2) -1/x2 = lim x® 0+  e1/x = ¥,

so from the right side, the line x = 0 is a vertical asymptote. However, if you approach from the other side,

lim x® 0-  xe1/x

is not an indeterminate form at all, since

lim x® 0-  e1/x = 0,

because 1/x is headed to negative infinity, and so

lim x® 0-  xe1/x = 0

as well. So, the line x = 0 is a vertical asymptote on one side only, and the graph approaches the origin from the other side (although (0,0) is of course not on the graph).

As x® ±¥, then e^1/x® e⁰ = 1 , and so xe^1/x approaches ±¥ as x does. But, for x large, since that exponential term is nearly 1, the graph ought to look like a line, and in fact

lim x® ¥  xe1/x-(x+1) = lim x® ¥  x( e1/x-(1+1/x)) ,

which is an indeterminate form of type ¥·0 , so converting to 0/0 ,

lim x® ¥  xe1/x-x = lim x® ¥  ( e1/x-(1+1/x)) 1/x = lim x® ¥  e1/x(-1/x2)-(-1/x2) -1/x2 , using l¢Hopital¢s rule = lim x® ¥  e1/x-1 = 0,

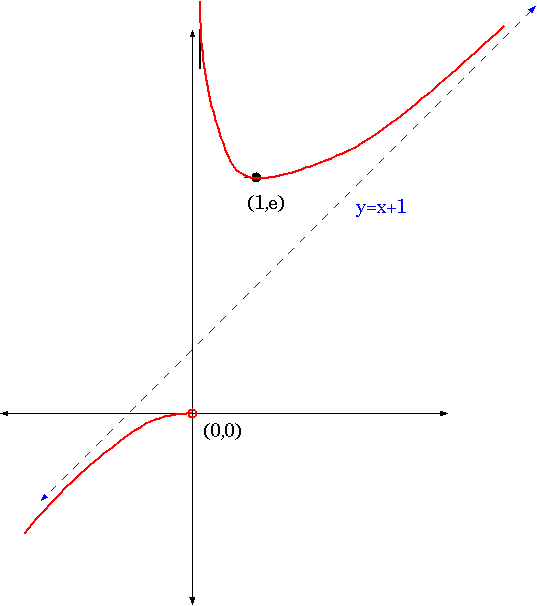
so that the graph has a slant-asymptote y = x+1 .

   Intercepts

Since 0 is not in the domain, there can be no y -intercept, even though there is a one-sided limit towards a point on the y -axis. For x ¹ 0 , though, there is no solution to f(x) = 0 , so that there are no x -intercepts, either.

   Increasing/decreasing, and critical points

f¢(x) = 1·e1/x+x·e1/x(-1/x2) = e1/x æ ç è 1- 1 x ö ÷ ø ,

so there is a critical point at x = 1 only, with critical value f(1) = e , and the curve is increasing ( f¢(x) > 0 ) for x > 1 , and for x < 0 . The curve is decreasing, f¢(x) < 0 , only when 0 < x < 1 .

   Concavity, and inflection points

f¢¢(x) = æ ç è e1/x æ ç è 1- 1 x ö ÷ ø ö ÷ ø ¢ = e1/x(-1/x2) æ ç è 1- 1 x ö ÷ ø +e1/x(+1/x2) = e1/x x2 æ ç è æ ç è 1 x -1 ö ÷ ø +1 ö ÷ ø = e1/x x3 ,

so there are no inflection points, the graph is concave up for x > 0 and concave down for x < 0 .

   Draw the graph

Use this information to draw a fair representation of the graph.

• The first information you plot are the asymptotes and ``tails'' [Link to ex8-1.gif]
• Then find intercepts and endpoints. But there are none for this graph.
• Then plot the critical points (and values). I always mark it with a horizontal line to remind myself that it is a critical point. [Link to ex8-2.gif]
• Plot the inflection points. None of these, at least
• Then fill in the graph, connecting the dots and making sure that the graph you draw is increasing/decreasing where the number line indicates it should be, and concave-up or concave-down where the sign of the second derivative indicates. [Link to ex8-3.gif]

[Each of these items should trigger the appearance of a new drawing with that information added.]

Copyright (c) 2000 by David L. Johnson.